Поверхность Хеннеберга
Поверхность Хеннеберга — неориентируемая минимальная поверхность[1], названная именем немецкого математика Лебрехта Хенненберга.
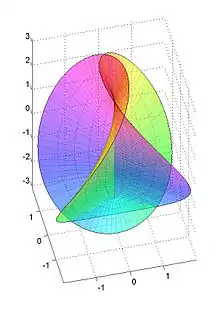
Поверхность Хеннеберга.
Поверхность имеет параметрические уравнения
и может быть описана как алгебраическая поверхность 15-го порядка[2]. Её можно рассматривать как погружение проколотой проективной плоскости[3]. До 1981 года поверхность была единственной известной неориентируемой минимальной поверхностью[4].
Поверхность содержит полукубическую параболу («параболу Нейла») и может быть получена решением соответствующей задачи Бьёрлинга[5][6].
Примечания
- Henneberg, 1875.
- Weisstein, Eric W. "Henneberg's Minimal Surface." From MathWorld—A Wolfram Web Resource. http://mathworld.wolfram.com/HennebergsMinimalSurface.html
- Dierkes, Hildebrandt, Sauvigny, 2010.
- de Oliveira, 1986.
- Henneberg, 1876, с. 66–70.
- Fung, 2004.
Литература
- L. Henneberg. Über salche minimalfläche, welche eine vorgeschriebene ebene curve sur geodätishen line haben. — Zürich: Eidgenössisches Polythechikum, 1875. — (Doctoral Dissertation).
- M. Elisa G. G. de Oliveira. Some New Examples of Nonorientable Minimal Surfaces // Proceedings of the American Mathematical Society. — 1986. — Декабрь (т. 98, № 4).
- Ulrich Dierkes, Stefan Hildebrandt, Friedrich Sauvigny. Minimal Surfaces 1. — Springer, 2010. — Т. 339. — (Grundlehren der mathematischen Wissenschaften). — ISBN 978-3-642-11697-1.
- L. Henneberg. Über diejenige minimalfläche, welche die Neil'sche Paralee zur ebenen geodätischen line hat // Vierteljschr Natuforsch, Ges.. — Zürich, 1876. — Вып. 21.
- Kai-Wing Fung. Minimal Surfaces as Isotropic Curves in C3: Associated minimal surfaces and the Björling's problem. — 2004. — (MIT BA Thesis).
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.
