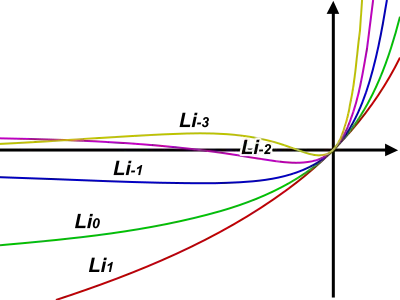
Полилогарифм

Полилогарифм — специальная функция, обозначаемая $\operatorname {Li} _{s}(z)$ и определяемая как бесконечный степенной ряд

\operatorname {Li} _{s}(z)=\sum _{k=1}^{\infty }{z^{k} \over k^{s}},

где s и z — комплексные числа, причём $|z|<1$ . Для иных z делается обобщение с помощью аналитического продолжения.

Карта высот полилогарифма на комплексной плоскости
$\operatorname {Li} _{-3}(z)$
$\operatorname {Li} _{-2}(z)$
$\operatorname {Li} _{-1}(z)$
$\operatorname {Li} _{0}(z)$
$\operatorname {Li} _{1}(z)$
$\operatorname {Li} _{2}(z)$
$\operatorname {Li} _{3}(z)$

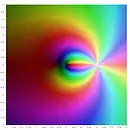
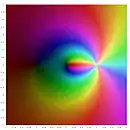
Частным случаем является $s=1$ , при котором $\operatorname {Li} _{1}(z)=-\ln(1-z)$ . Функции $\operatorname {Li} _{2}(z)$ и $\operatorname {Li} _{3}(z)$ получили названия дилогарифма и трилогарифма соответственно. Для полилогарифмов различных порядков справедливо соотношение

\operatorname {Li} _{s+1}(z)=\int _{0}^{z}{\frac {\operatorname {Li} _{s}(t)}{t}}\,\mathrm {d} t.

Альтернативными определениями полилогарифма являются интегралы Ферми — Дирака и Бозе — Эйнштейна.

Частные значения

\operatorname {Li} _{1}({\tfrac {1}{2}})=\ln 2

\operatorname {Li} _{2}({\tfrac {1}{2}})={\tfrac {1}{12}}\pi ^{2}-{\tfrac {1}{2}}(\ln 2)^{2}

\operatorname {Li} _{3}({\tfrac {1}{2}})={\tfrac {1}{6}}(\ln 2)^{3}-{\tfrac {1}{12}}\pi ^{2}\ln 2+{\tfrac {7}{8}}\,\zeta (3)\,

(где

\,\zeta (3)\,

— постоянная Апери)

\operatorname {Li} _{1}(z)=-\ln(1-z)

\operatorname {Li} _{0}(z)={z \over 1-z}

\operatorname {Li} _{-1}(z)={z \over (1-z)^{2}}

\operatorname {Li} _{-2}(z)={z\,(1+z) \over (1-z)^{3}}

\operatorname {Li} _{-3}(z)={z\,(1+4z+z^{2}) \over (1-z)^{4}}

\operatorname {Li} _{-4}(z)={z\,(1+z)(1+10z+z^{2}) \over (1-z)^{5}}\,.

Литература

Abel, N.H. Œuvres complètes de Niels Henrik Abel − Nouvelle édition, Tome II (фр.) / Sylow, L.; Lie, S.. — Christiania [Oslo]: Grøndahl & Søn, 1881. — С. 189—193. (this 1826 manuscript was only published posthumously.)
Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables (англ.). — New York: Dover Publications, 1972. — ISBN 0-486-61272-4.
Bailey, D.H.; Borwein, P.B.; Plouffe, S. On the Rapid Computation of Various Polylogarithmic Constants (англ.) // Mathematics of Computation : journal. — 1997. — April (vol. 66, no. 218). — P. 903—913. — doi:10.1090/S0025-5718-97-00856-9.
Bailey, D.H. & Broadhurst, D.J. (June 20, 1999), A Seventeenth-Order Polylogarithm Ladder, arΧiv:math.CA/9906134 [math.CA]

Berndt, B.C. Ramanujan's Notebooks, Part IV (неопр.). — New York: Springer-Verlag, 1994. — С. 323—326. — ISBN 0-387-94109-6.
Boersma, J.; Dempsey, J.P. On the evaluation of Legendre's chi-function (англ.) // Mathematics of Computation : journal. — 1992. — Vol. 59, no. 199. — P. 157—163. — doi:10.2307/2152987. — .
Borwein, J.M.; Bradley, D.M.; Broadhurst, D.J.; Lisonek, P. Special Values of Multiple Polylogarithms (англ.) // Transactions of the American Mathematical Society. — 2001. — Vol. 353, no. 3. — P. 907—941. — doi:10.1090/S0002-9947-00-02616-7.
Clunie, J. On Bose-Einstein functions (англ.) // Proceedings of the Physical Society, Section A : journal. — 1954. — Vol. 67, no. 7. — P. 632—636. — doi:10.1088/0370-1298/67/7/308.
Cohen, H.; Lewin, L.; Zagier, D. A Sixteenth-Order Polylogarithm Ladder (неопр.) // Experimental Mathematics. — 1992. — Т. 1, № 1. — С. 25—34. Архивировано 1 марта 2012 года.
Coxeter, H.S.M. The functions of Schläfli and Lobatschefsky (неопр.) // Quarterly Journal of Mathematics (Oxford). — 1935. — Т. 6, № 1. — С. 13—29. — doi:10.1093/qmath/os-6.1.13.
Cvijovic, D.; Klinowski, J. Continued-fraction expansions for the Riemann zeta function and polylogarithms (англ.) // Proceedings of the American Mathematical Society : journal. — 1997. — Vol. 125, no. 9. — P. 2543—2550. — doi:10.1090/S0002-9939-97-04102-6.
Cvijovic, D. New integral representations of the polylogarithm function (англ.) // Proceedings of the Royal Society (London), Series A : journal. — 2007. — Vol. 463, no. 2080. — P. 897—905. — doi:10.1098/rspa.2006.1794.
Erdélyi, A.; Magnus, W.; Oberhettinger, F.; Tricomi, F.G. Higher Transcendental Functions, Vol. 1 (неопр.). — New York: Krieger, 1981.
Fornberg, B.; Kölbig, K.S. Complex zeros of the Jonquiére or polylogarithm function (англ.) // Mathematics of Computation : journal. — 1975. — Vol. 29, no. 130. — P. 582—599. — doi:10.2307/2005579. — .
GNU Scientific Library. Reference Manual (неопр.) (2010). Дата обращения: 13 июня 2010. Архивировано 14 мая 2012 года.
Gradshteyn, I.S.; Ryzhik, I.M. Tables of Integrals, Series, and Products (англ.). — 4th. — New York: Academic Press, 1980. — ISBN 0-12-294760-6.
Guillera, J.; Sondow, J. Double integrals and infinite products for some classical constants via analytic continuations of Lerch's transcendent (англ.) // The Ramanujan Journal : journal. — 2008. — Vol. 16, no. 3. — P. 247—270. — doi:10.1007/s11139-007-9102-0. — arXiv:math.NT/0506319.
Hain, R.M. (March 25, 1992), Classical polylogarithms, arΧiv:alg-geom/9202022 [alg-geom]

Jahnke, E.; Emde, F. Tables of Functions with Formulae and Curves (англ.). — 4th. — New York: Dover Publications, 1945.
Jonquière, A. Note sur la série $\scriptstyle \sum _{n=1}^{\infty }{\frac {x^{n}}{n^{s}}}$ (фр.) // Bulletin de la Société Mathématique de France. — 1889. — Т. 17. — С. 142—152.
Kölbig, K.S.; Mignaco, J.A.; Remiddi, E. On Nielsen's generalized polylogarithms and their numerical calculation (англ.) // BIT : journal. — 1970. — Vol. 10. — P. 38—74. — doi:10.1007/BF01940890.
Kirillov, A.N. Dilogarithm identities (англ.) // Progress of Theoretical Physics Supplement : journal. — 1995. — Vol. 118. — P. 61—142. — doi:10.1143/PTPS.118.61. — arXiv:hep-th/9408113.
Lewin, L. Dilogarithms and Associated Functions (неопр.). — London: Macdonald, 1958.
Lewin, L. Polylogarithms and Associated Functions (неопр.). — New York: North-Holland, 1981. — ISBN 0-444-00550-1.
Lewin, L. (Ed.). Structural Properties of Polylogarithms (неопр.). — Providence, RI: Amer. Math. Soc., 1991. — Т. 37. — (Mathematical Surveys and Monographs). — ISBN 0-8218-1634-9.
Markman, B. The Riemann Zeta Function (неопр.) // BIT. — 1965. — Т. 5. — С. 138—141.
Maximon, L.C. The Dilogarithm Function for Complex Argument (неопр.) // Proceedings of the Royal Society (London), Series A. — 2003. — Т. 459, № 2039. — С. 2807—2819. — doi:10.1098/rspa.2003.1156.
McDougall, J.; Stoner, E.C. The computation of Fermi-Dirac functions (неопр.) // Philosophical Transactions of the Royal Society (London), Series A. — 1938. — Т. 237, № 773. — С. 67—104. — doi:10.1098/rsta.1938.0004.
Nielsen, N. Der Eulersche Dilogarithmus und seine Verallgemeinerungen (нем.) // Nova Acta Leopoldina. — Halle – Leipzig, Germany: Kaiserlich-Leopoldinisch-Carolinische Deutsche Akademie der Naturforscher, 1909. — Т. XC, № 3. — С. 121—212.
Prudnikov, A.P.; Marichev, O.I.; Brychkov, Yu.A. Integrals and Series, Vol. 3: More Special Functions (англ.). — Newark, NJ: Gordon and Breach, 1990. — ISBN 2-88124-682-6. (see § 1.2, «The generalized zeta function, Bernoulli polynomials, Euler polynomials, and polylogarithms», p. 23.)
Robinson, J.E. Note on the Bose-Einstein integral functions (неопр.) // Physical Review, Series 2. — 1951. — Т. 83, № 3. — С. 678—679. — doi:10.1103/PhysRev.83.678.
Rogers, L.J. On function sum theorems connected with the series $\scriptstyle \sum _{n=1}^{\infty }{\frac {x^{n}}{n^{2}}}$ (англ.) // Proceedings of the London Mathematical Society (2) : journal. — 1907. — Vol. 4, no. 1. — P. 169—189. — doi:10.1112/plms/s2-4.1.169.
Erwin Schrödinger. Statistical Thermodynamics (неопр.). — 2nd. — Cambridge, UK: Cambridge University Press, 1952.
Truesdell, C. On a function which occurs in the theory of the structure of polymers (англ.) // Annals of Mathematics, Series 2 : journal. — 1945. — Vol. 46, no. 1. — P. 144—157. — doi:10.2307/1969153. — .
Vepstas, L. (February 2007), An efficient algorithm for accelerating the convergence of oscillatory series, useful for computing the polylogarithm and Hurwitz zeta functions, arΧiv:math.CA/0702243 [math.CA]

Whittaker, E.T.; Watson, G.N. A Course of Modern Analysis (неопр.). — 4th. — Cambridge, UK: Cambridge University Press, 1952.
Wood, D.C. The Computation of Polylogarithms. Technical Report 15-92* (неопр.) (PS). Canterbury, UK: University of Kent Computing Laboratory (June 1992). Дата обращения: 1 ноября 2005. Архивировано 14 мая 2012 года.
Zagier, D. (1989). «The dilogarithm function in geometry and number theory». Number Theory and Related Topics: papers presented at the Ramanujan Colloquium, Bombay, 1988 12: 231–249, Bombay: Tata Institute of Fundamental Research and Oxford University Press. (also appeared as «The remarkable dilogarithm» in Journal of Mathematical and Physical Sciences 22 (1988), pp. 131—145, and as Chapter I of (Zagier 2007).)
Zagier, D. Frontiers in Number Theory, Physics, and Geometry II – On Conformal Field Theories, Discrete Groups and Renormalization (англ.) / Cartier, P.; Julia, B.; Moussa, P.; Vanhove, P.. — Berlin: Springer-Verlag, 2007. — P. 3—65. — ISBN 978-3-540-30307-7.

Ссылки

Weisstein, Eric W. Polylogarithm (англ.) на сайте Wolfram MathWorld.
Weisstein, Eric W. Dilogarithm (англ.) на сайте Wolfram MathWorld.

This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.